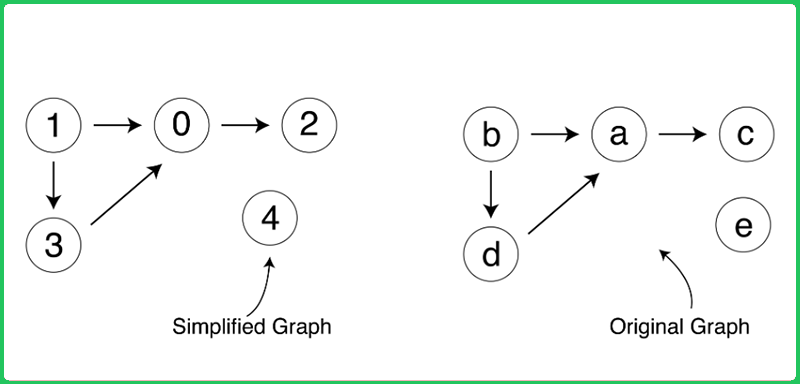
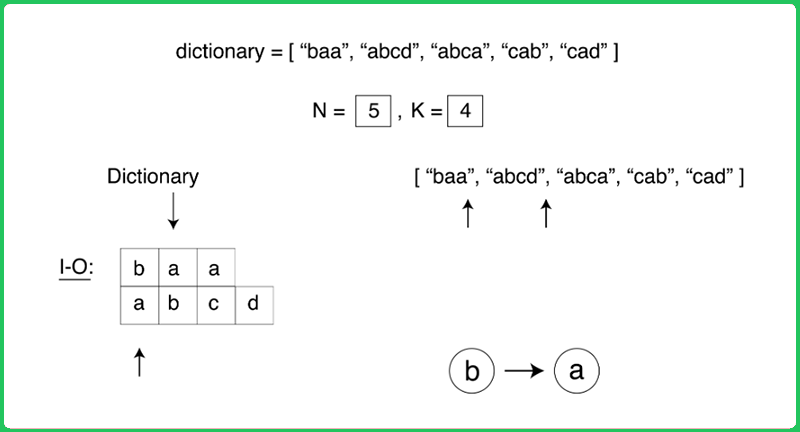
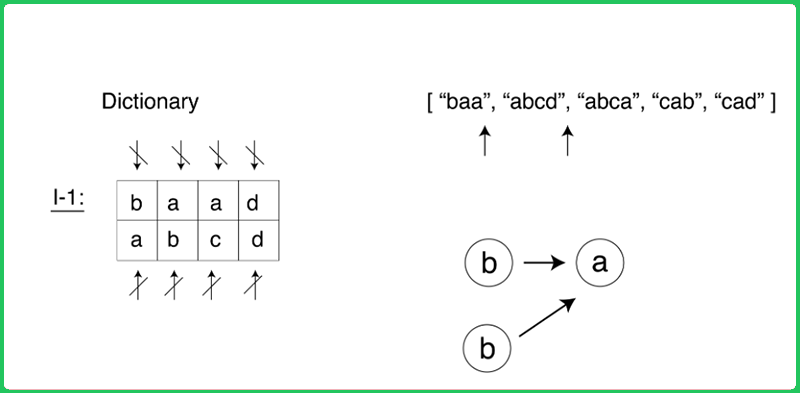
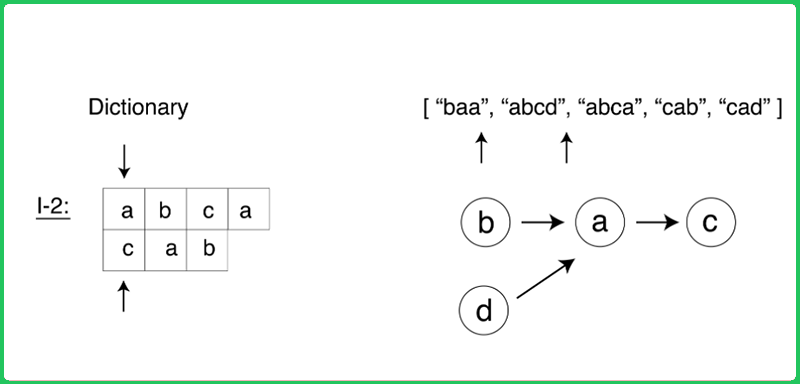
123456789101112131415161718192021222324252627282930313233343536373839404142434445464748495051525354555657585960616263646566676869707172737475767778798081828384858687888990919293949596979899100101102103104105106107108109110111112113114115116117118119120121122123124125126127
#include <bits/stdc++.h>
using namespace std;
class Solution {
private:
/* Function to return the topological
sorting of given graph */
vector<int> topoSort(int V, vector<int> adj[]) {
// To store the In-degrees of nodes
vector<int> inDegree(V, 0);
// Update the in-degrees of nodes
for (int i = 0; i < V; i++) {
for(auto it : adj[i]) {
// Update the in-degree
inDegree[it]++;
}
}
// To store the result
vector<int> ans;
// Queue to facilitate BFS
queue<int> q;
// Add the nodes with no in-degree to queue
for(int i=0; i<V; i++) {
if(inDegree[i] == 0) q.push(i);
}
// Until the queue is empty
while(!q.empty()) {
// Get the node
int node = q.front();
// Add it to the answer
ans.push_back(node);
q.pop();
// Traverse the neighbours
for(auto it : adj[node]) {
// Decrement the in-degree
inDegree[it]--;
/* Add the node to queue if
its in-degree becomes zero */
if(inDegree[it] == 0) q.push(it);
}
}
// Return the result
return ans;
}
public:
/* Function to determine order of
letters based on alien dictionary */
string findOrder(string dict[], int N, int K) {
// Initialise a graph of K nodes
vector<int> adj[K];
// Compare the consecutive words
for(int i=0; i < N-1; i++) {
string s1 = dict[i];
string s2 = dict[i + 1];
int len = min(s1.size(), s2.size());
/* Compare the pair of strings letter by
letter to identify the differentiating letter */
for (int ptr = 0; ptr < len; ptr++) {
// If the differentiating letter is found
if (s1[ptr] != s2[ptr]) {
// Add the edge to the graph
adj[s1[ptr] - 'a'].push_back(s2[ptr] - 'a');
break;
}
}
}
/* Get the topological sort
of the graph formed */
vector<int> topo = topoSort(K, adj);
// To store the answer
string ans;
for(int i=0; i < K; i++) {
// Add the letter to the result
ans.push_back('a' + topo[i]);
ans.push_back(' ');
}
// Return the answer
return ans;
}
};
int main() {
int N = 5, K = 4;
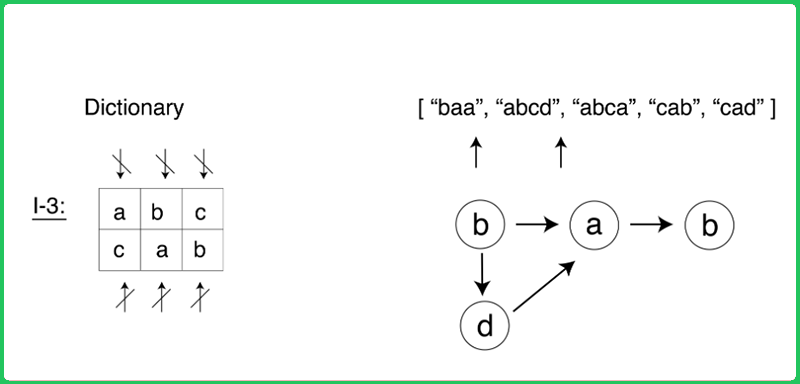
string dict[N] = {
"baa","abcd","abca","cab","cad"
};
/* Creating an instance of
Solution class */
Solution sol;
/* Function call to determine order of
letters based on alien dictionary */
string ans = sol.findOrder(dict, N, K);
// Output
cout << "The order to characters as per alien dictionary is: " << ans;
return 0;
}
123456789101112131415161718192021222324252627282930313233343536373839404142434445464748495051525354555657585960616263646566676869707172737475767778798081828384858687888990919293949596979899100101102103104105106107108109110111112113114115116117118119120121122
import java.util.*;
class Solution {
/* Function to return the topological
sorting of given graph */
private List<Integer> topoSort(int V, List<Integer>[] adj) {
// To store the In-degrees of nodes
int[] inDegree = new int[V];
// Update the in-degrees of nodes
for (int i = 0; i < V; i++) {
for (int it : adj[i]) {
// Update the in-degree
inDegree[it]++;
}
}
// To store the result
List<Integer> ans = new ArrayList<>();
// Queue to facilitate BFS
Queue<Integer> q = new LinkedList<>();
// Add the nodes with no in-degree to queue
for (int i = 0; i < V; i++) {
if (inDegree[i] == 0) q.add(i);
}
// Until the queue is empty
while (!q.isEmpty()) {
// Get the node
int node = q.poll();
// Add it to the answer
ans.add(node);
// Traverse the neighbours
for (int it : adj[node]) {
// Decrement the in-degree
inDegree[it]--;
/* Add the node to queue if
its in-degree becomes zero */
if (inDegree[it] == 0) q.add(it);
}
}
// Return the result
return ans;
}
/* Function to determine order of
letters based on alien dictionary */
public String findOrder(String[] dict, int N, int K) {
// Initialise a graph of K nodes
List<Integer>[] adj = new ArrayList[K];
for (int i = 0; i < K; i++) adj[i] = new ArrayList<>();
// Compare the consecutive words
for (int i = 0; i < N - 1; i++) {
String s1 = dict[i];
String s2 = dict[i + 1];
int len = Math.min(s1.length(), s2.length());
/* Compare the pair of strings letter by
letter to identify the differentiating letter */
for (int ptr = 0; ptr < len; ptr++) {
// If the differentiating letter is found
if (s1.charAt(ptr) != s2.charAt(ptr)) {
// Add the edge to the graph
adj[s1.charAt(ptr) - 'a'].add(s2.charAt(ptr) - 'a');
break;
}
}
}
/* Get the topological sort
of the graph formed */
List<Integer> topo = topoSort(K, adj);
// To store the answer
StringBuilder ans = new StringBuilder();
for (int i = 0; i < K; i++) {
// Add the letter to the result
ans.append((char) ('a' + topo.get(i)));
ans.append(' ');
}
// Return the answer
return ans.toString();
}
public static void main(String[] args) {
int N = 5, K = 4;
String[] dict = {
"baa", "abcd", "abca", "cab", "cad"
};
/* Creating an instance of
Solution class */
Solution sol = new Solution();
/* Function call to determine order of
letters based on alien dictionary */
String ans = sol.findOrder(dict, N, K);
// Output
System.out.println("The order to characters as per alien dictionary is: " + ans);
}
}
123456789101112131415161718192021222324252627282930313233343536373839404142434445464748495051525354555657585960616263646566676869707172737475767778798081828384858687888990919293949596979899100101
from collections import deque
class Solution:
# Function to return the topological
# sorting of given graph
def topoSort(self, V, adj):
# To store the In-degrees of nodes
inDegree = [0] * V
# Update the in-degrees of nodes
for i in range(V):
for it in adj[i]:
# Update the in-degree
inDegree[it] += 1
# To store the result
ans = []
# Queue to facilitate BFS
q = deque()
# Add the nodes with no in-degree to queue
for i in range(V):
if inDegree[i] == 0:
q.append(i)
# Until the queue is empty
while q:
# Get the node
node = q.popleft()
# Add it to the answer
ans.append(node)
# Traverse the neighbours
for it in adj[node]:
# Decrement the in-degree
inDegree[it] -= 1
# Add the node to queue if its in-degree becomes zero
if inDegree[it] == 0:
q.append(it)
# Return the result
return ans
# Function to determine order of
# letters based on alien dictionary
def findOrder(self, dict, N, K):
# Initialise a graph of K nodes
adj = [[] for _ in range(K)]
# Compare the consecutive words
for i in range(N - 1):
s1 = dict[i]
s2 = dict[i + 1]
length = min(len(s1), len(s2))
# Compare the pair of strings letter by
# letter to identify the differentiating letter
for ptr in range(length):
# If the differentiating letter is found
if s1[ptr] != s2[ptr]:
# Add the edge to the graph
adj[ord(s1[ptr]) - ord('a')].append(ord(s2[ptr]) - ord('a'))
break
# Get the topological sort
# of the graph formed
topo = self.topoSort(K, adj)
# To store the answer
ans = ""
for i in range(K):
# Add the letter to the result
ans += chr(ord('a') + topo[i])
ans += ' '
# Return the answer
return ans
# Example usage
N = 5
K = 4
dict = [
"baa", "abcd", "abca", "cab", "cad"
]
sol = Solution()
ans = sol.findOrder(dict, N, K)
# Output
print("The order to characters as per alien dictionary is:", ans)
123456789101112131415161718192021222324252627282930313233343536373839404142434445464748495051525354555657585960616263646566676869707172737475767778798081828384858687888990919293949596979899100101102103104105106107108109110111112113
class Solution {
/* Function to return the topological
sorting of given graph */
topoSort(V, adj) {
// To store the In-degrees of nodes
let inDegree = new Array(V).fill(0);
// Update the in-degrees of nodes
for (let i = 0; i < V; i++) {
for (let it of adj[i]) {
// Update the in-degree
inDegree[it]++;
}
}
// To store the result
let ans = [];
// Queue to facilitate BFS
let q = [];
// Add the nodes with no in-degree to queue
for (let i = 0; i < V; i++) {
if (inDegree[i] == 0) q.push(i);
}
// Until the queue is empty
while (q.length > 0) {
// Get the node
let node = q.shift();
// Add it to the answer
ans.push(node);
// Traverse the neighbours
for (let it of adj[node]) {
// Decrement the in-degree
inDegree[it]--;
/* Add the node to queue if
its in-degree becomes zero */
if (inDegree[it] == 0) q.push(it);
}
}
// Return the result
return ans;
}
/* Function to determine order of
letters based on alien dictionary */
findOrder(dict, N, K) {
// Initialise a graph of K nodes
let adj = new Array(K).fill().map(() => []);
// Compare the consecutive words
for (let i = 0; i < N - 1; i++) {
let s1 = dict[i];
let s2 = dict[i + 1];
let len = Math.min(s1.length, s2.length);
/* Compare the pair of strings letter by
letter to identify the differentiating letter */
for (let ptr = 0; ptr < len; ptr++) {
// If the differentiating letter is found
if (s1[ptr] != s2[ptr]) {
// Add the edge to the graph
adj[s1.charCodeAt(ptr) - 'a'.charCodeAt(0)].push(s2.charCodeAt(ptr) - 'a'.charCodeAt(0));
break;
}
}
}
/* Get the topological sort
of the graph formed */
let topo = this.topoSort(K, adj);
// To store the answer
let ans = '';
for (let i = 0; i < K; i++) {
// Add the letter to the result
ans += String.fromCharCode('a'.charCodeAt(0) + topo[i]);
ans += ' ';
}
// Return the answer
return ans;
}
}
// Example usage
const N = 5;
const K = 4;
const dict = [
"baa", "abcd", "abca", "cab", "cad"
];
const sol = new Solution();
const ans = sol.findOrder(dict, N, K);
// Output
console.log("The order to characters as per alien dictionary is:", ans);